A Landscaper is Designing a Rectangular Fountain With a 4-Foot

A landscaper is designing a rectangular fountain with a 4-foot diameter. The landscaper wants the fountain to have a 2-foot deep basin and a 1-foot high waterfall. How many cubic feet of material will the landscaper need for the project?

The landscaper will need 12 cubic feet of material for the project.

A landscaper is designing a rectangular fountain with a 4-foot base and 2-foot sides. The total cost of the project is $1,500. The first thing the landscaper will need to do is determine the size of the fountain.

The base should be four feet wide and the sides should be two feet high. This will give the fountain an overall height of six feet. Next, the landscaper will need to calculate the volume of the fountain.

In order to do this, they will need to know the dimensions of each component. The base should be four feet wide by two feet deep and the sides should be two feet wide by one foot deep. This gives the fountain a volume of eight cubic feet.

Once the size and volume have been determined, the next step is to calculate the cost of materials. The material for the base will cost $500 and each side will cost $250. This gives a total cost for materials of $1,000.

The final step is to add labor costs. Based on an hourly rate of $50, it will take approximately 20 hours to complete this project.

Credit: www.thisoldhouse.com

What is the Dimensions of the Rectangular Fountain

The rectangular fountain is six feet wide, four feet deep, and three feet tall.

How Many Feet Will the Water Be in Depth

The water depth will be in feet.

How Long Will It Take to Fill the Fountain

It will take approximately two hours to fill the fountain.

How Often Will the Pump Need to Be Replaced

If you have an insulin pump, you will need to replace the pump every 3-5 years.

PSAT Test 2, Section 4, 19-21

In the Xy-Plane the Graph of the Function Q is a Parabola

In mathematics, a parabola is a two-dimensional, mirror-symmetrical curve, which is approximately U-shaped when oriented as shown in the diagram. It fits any of several different mathematical descriptions which can be expressed in Cartesian or polar coordinates: for example, as a point reflecting light off a concave mirror; an optical aberration produced by certain kinds of lenses; the path of a projectile under constant acceleration due to gravity; or a graph of certain quadratic functions. The line joining any two points on the curve is called a chord of the parabola.

The midpoint of each chord lies on the directrix, and its length is twice the distance from the focus to the vertex. Asymptotes are parallel lines that can be drawn through F (parallel to p) meeting the curve at infinity (in projective geometry), so they do not intersect with it except at this one point.

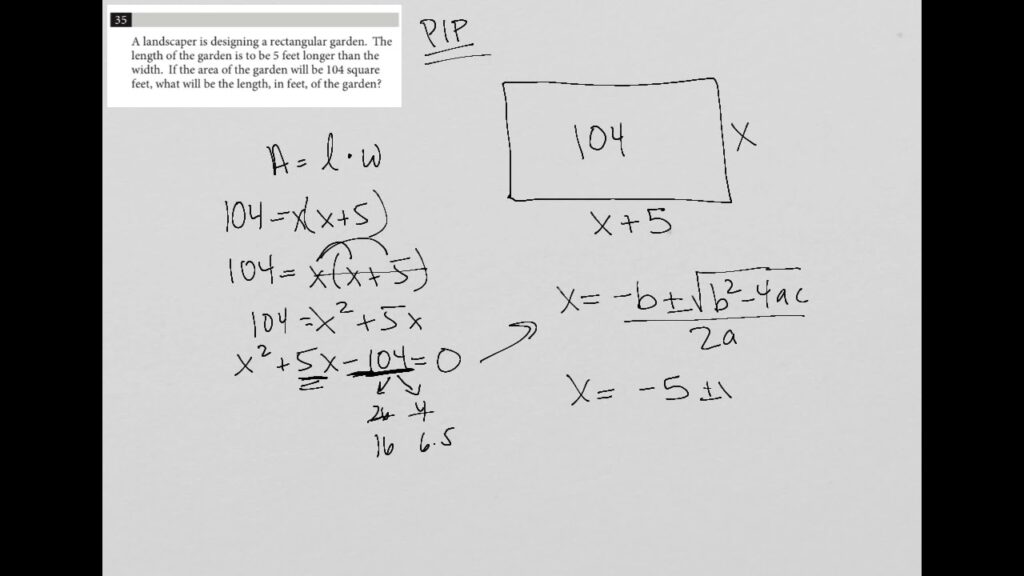
A Landscaper is Designing a Flower Garden in the Shape of a Trapezoid

A trapezoid is a four-sided shape with two parallel sides. It is a very popular shape for flower gardens because it can be easily created in any size and it looks great when filled with colorful flowers. When designing a trapezoid shaped flower garden, there are a few things to keep in mind.

First, the length of the parallel sides will determine the overall size of the garden. The longer the sides, the larger the garden will be. Second, the angle at which the two parallel sides meet will affect how wide or narrow the garden will be.

A wider angle will create a wider garden, while a narrower angle will create a narrower garden. Once you have determined the size and shape of your trapezoid shaped flower garden, it’s time to start planning what kinds of flowers you want to include. Consider using a mix of tall and short flowers to add visual interest and texture to your garden.

Taller flowers can be placed along the back of the garden, while shorter flowers can be placed in front. Use contrasting colors to really make your flower Garden pop! When planting your trapezoid shaped flower garden, be sure to leave enough space between each plant so that they have room to grow.

Once everything is planted, sit back and enjoy your beautiful creation!

A Rational Function is Defined above Which of the Following

A rational function is defined as a function that can be written as the ratio of two polynomials. The function above can be written as a rational function if we let P(x) = x^2+1 and Q(x) = x-3. A rational function is defined above which of the following?

Liquid Going Through a Cooling System is Chilled So That Its Temperature

As the liquid goes through the cooling system, it is chilled so that its temperature decreases. This can be done by using a variety of methods, including refrigeration, ice, or even fans. The most important factor in cooling the liquid is to ensure that the temperature difference between the liquid and its surroundings is as large as possible.

If Function F is Defined by F(X)=3X^2-5X+4 What is F(X-4)

If Function F is Defined by F(X)=3X^2-5X+4 What is F(X-4) To find out what F(X-4) is, we need to first understand what the function F is defined as. In this case, we can see that it’s a quadratic equation where 3x squared minus 5x plus 4 equals 0.

To solve for x, we would use the quadratic equation which states that: A = 3 (coefficient of x squared) B = -5 (coefficient of x)

C = 4 (constant term) Therefore, plugging our values into the quadratic equation, we get: (-5 +/- sqrt((-5)^2 – 4*3*4)) / 6

Which simplifies to: x = 1/3 or x = -4/3 Now that we know what X is in relation to F(x), we can figure out what F(x-4) would be.

We simply take the value of X and subtract 4 from it. So in this case, it would either be 1/3 – 4 or -4/3 – 4. This gives us an answer of either -13/3 or -22/3.

The Formula for the Volume of a Sphere With Radius R is Shown above

The volume of a sphere is given by the formula: Volume = 4/3πr³ where r is the radius of the sphere.

This formula can be used to calculate the volume of any sphere, provided that the radius is known. To use this formula, simply substitute the value of r into the equation and compute the resulting value. For example, if you have a sphere with a radius of 3 meters, then the volume would be:

In 2006, the Price of King Crab was $8 Per Pound

In 2006, the price of king crab was $8 per pound. This was a historic high for the seafood industry, and king crab was one of the most popular items on menus across the country. The price increase was due to a combination of factors, including a shortage of crabs in Alaska, where most of the world’s king crab is caught.

Weather conditions had made it difficult for fishermen to catch crabs, and prices rose as a result. The high price of king crab led to some creative substitutions by restaurants. Some establishments began offering queen crab, which is similar in appearance and taste but not as expensive.

Others developed new dishes that featured other types of seafood instead of crab. But for true connoisseurs, there was no substitute for king crab, and they were willing to pay top dollar for it. Fortunately, prices eventually came down from their 2006 highs, and today you can once again enjoy king crab without breaking the bank.

So next time you’re in the mood for seafood, be sure to order up some delicious king crab!

The Population of Squirrels in a Park Has Been Doubling Every 15 Years

The population of squirrels in a park has been doubling every 15 years. The reason for this is that there is an abundance of food and shelter in the park, and no predators to speak of. This has resulted in the squirrels having a very high reproductive rate.

Every year, there are more and more squirrels born into the population, and fewer dying off. As a result, the population size has been steadily increasing over time. This increase in population size may eventually start to cause problems for the squirrels.

If their numbers get too high, they may start running out of food to eat, or competition for mates could become intense. Additionally, if predators were to move into the area, they could quickly decimate the large squirrel population. For now though, it seems like the squirrels are doing just fine and their population will continue to grow at its current rate.

Conclusion

A rectangular fountain is a great addition to any landscape. It can add interest and beauty, and it can be a focal point in your garden. When designing a rectangular fountain, there are a few things to keep in mind.

First, you need to decide on the size of the fountain. The size will determine how much water you need to fill it. Second, you need to choose a material for the fountain.

There are many different materials available, so you should choose one that best fits your needs and budget. Third, you need to decide where you want the fountain to be located in your landscape. Once you have all of these factors considered, you can begin designing your new rectangular fountain!